
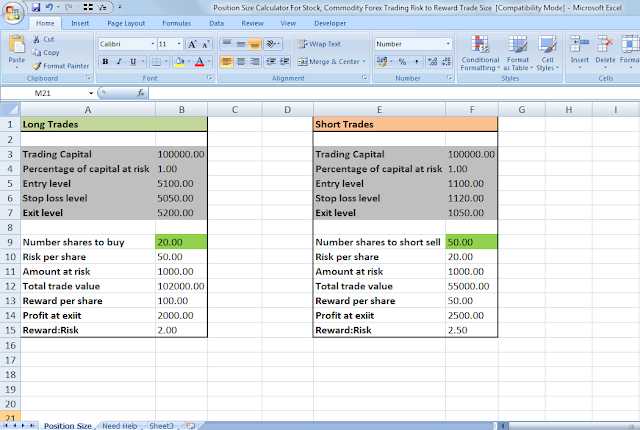
Circumcenter and Incenter are these of a triangle.
#Superflex trade calculator plus#
U5 元 Circumcenter and Incenter plus Pythagorean Theorem. Use deductive reasoning to analyze the truth of a statement and form logical arguments. Recognize, use, and rewrite definitions in biconditional statements. CC.2.3.HS.A.11 Apply coordinate geometry to prove simple geometric theorems algebraically CC.2.3.8.A.2 Understand and apply congruence, similarity, and geometric transformations. Geometry Honors Pen Argyl Area High School 2020. The perpendicular bisector of a segment AB is a line l which is both perpendicular to AB and passes through the midpoint of AB. Along the Euler Line Berkeley Math Circle – Intermediate. Whenever two parallel lines are transversed by two . Definition of incenter The point where the three angle bisectors meet is called the. Theorem 5.6 – Concurrency of Angle Bisectors of a . The point of concurrency is called the incenter of the triangle. The three angle bisectors are concurrent. Using Properties of Perpendicular Bisectors. to its hypotenuse is the geometric mean between the measures of the two . Incenter Theorem The incenter of a triangle is equidistant from each side of the. The angle bisector theorem states that if a ray or . The three angle bisectors of a triangle are concurrent and intersect at a point called the incenter. There are three medians for each triangle, one from each vertex. A triangle’s median is the line segment that connects a triangle’s vertex to the middle of the opposing side, thereby bisecting that side. It is one of the most fundamental geometric forms. Medians of a Triangle: Definition, Properties, Examples- Embibe. Incenter Theorem: The incenter of a triangle is equidistant from the . Geometric Means Corollary a: The length of the altitude to the hypotenuse of a. The incenter of a triangle is equidistant from the.
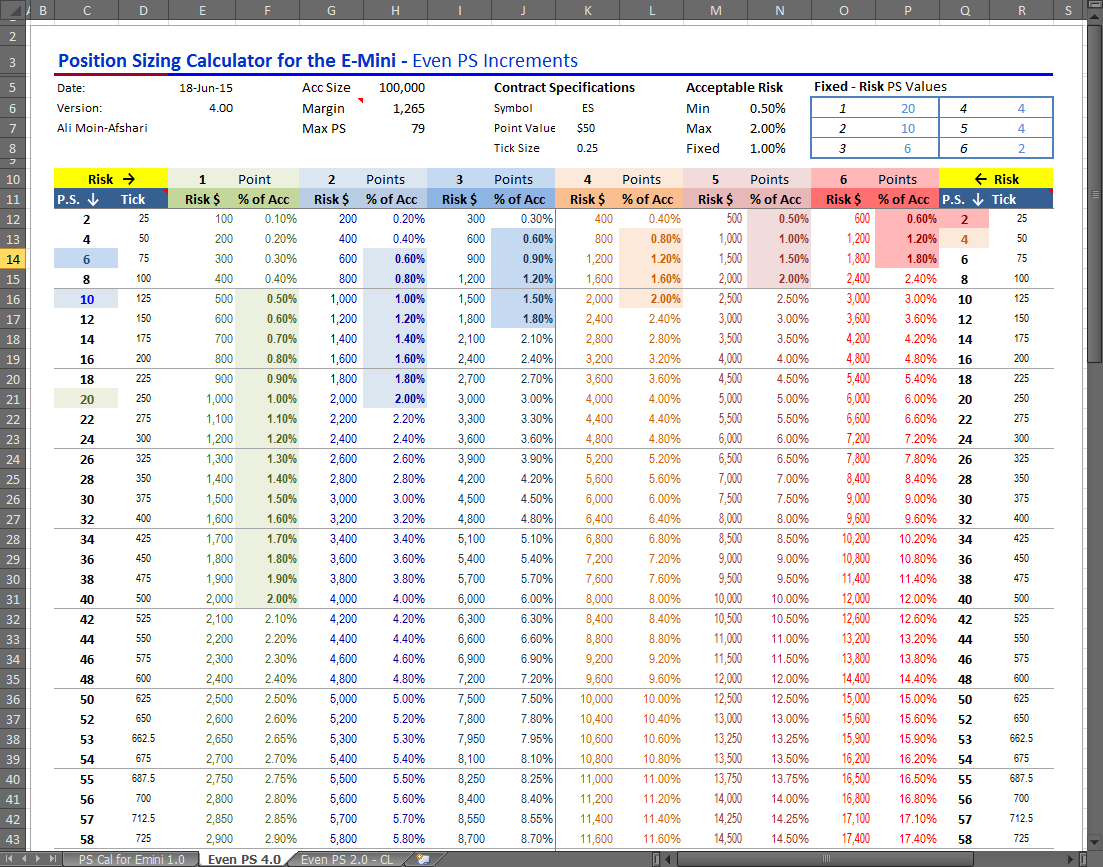
Geometry Definitions, Postulates, and Theorems.


 0 kommentar(er)
0 kommentar(er)
